2015-2016 Geometry INB Unit 10 (Circles) [#MTBoSBlaugust]

It's #MTBoSBlaugust 2016!  I'm challenging myself to blog daily throughout August, and you can too.  Join the fun!

See last year's INB pages from this unit here.

Last unit.  Here is the table of contents:

This year I had to review completing the square for circle equations.  You know how when you review something from the previous year, and your students all try to convince that they never learned it?  This time they may have been right.  I tried to review completing the square.  I failed because from what I could tell, they didn't learn it enough to review.  (From what I was told, these students didn't quite make it through the factoring unit.)  I made a booklet for the notes.  The front I tried to write out the steps and show an example step-by-step next to them.  Then there are four examples of just completing the square.  I accidentally included an example that lead to an answer with imaginary numbers.  We would have just skipped over that example in class, but we ended up skipping over all of these examples to be honest.  My class begged to just jump into the process they had to know for circle equations. *sigh*

 My tangent theorem page was different from last year.  I removed common tangents.  I'm considering adding it back in because what I'm realizing with Common Core is that nothing has really been removed, it's just been hidden in a vaguely worded standard.

I made my last booklet.  I combined central and inscribed angles into to one day with inscribed polygons.  Next year these will be two separate days.  I plan on making activities to help students better understand these topics, and separating them would help with allowing everything to sink in.

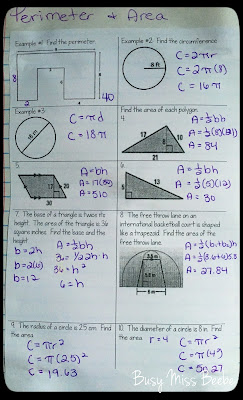
Lastly, we covered arc lengths and area of sectors.  Next year I need to add radians to this lesson.  I didn't realize that it was part of the standards this year, and it appeared on the Regents.  Also, I really want more examples for these topics. 

I'm so excited to start overhauling my notes for next year.  I really enjoy going through each unit and finding things that I could do better.  I hope my musings have helped you as well.

2015-2016 Geometry INB Unit 9 (Coordinate Geometry) [#MTBoSBlaugust]

It's #MTBoSBlaugust 2016!  I'm challenging myself to blog daily throughout August, and you can too.  Join the fun!

See last year's INB pages from this unit here.

This unit has also had a complete overhaul from last year.  Here is the table of contents:

We started by reviewing writing linear equations.  The one thing students seem to need the most practice on is solving for y when the equation is given in standard form.

Then we did the slope of parallel and perpendicular lines.  I kept this very simple.  I definitely want to give a discovery activity I have for this topic another try.

Then we combined the first two topics into Equations of Parallel & Perpendicular lines.

The next day we moved to Partitioning Segments.  I was so lost on this topic at first because I had to teach myself before I could teach this to my students.  I wrote a reflection on how my first time teaching it went.  I literally copied what I could find on Math Bits Notebook into the notes because I did not know how to articulate the concept.  I'm excited for teaching this again next year now that I know how to teach it.

Our next topic was the Distance Formula.  Next year I want to include grids into the notes so students have the tools necessary to use the Pythagorean Theorem if they wish.

The next day we had the lesson that wasn't.  Something else was going that day so it was a quick explanation, and a recommendation that students try to do two more examples on their own.  I was not quite sure about how to incorporate the Perpendicular Bisector Theorem, or if it was supposed to be included at all.  I used to teach students how to find the equation of the perpendicular bisector.  Next year I will teach how to find the equation, and then we will do this example of verifying the theorem.

Then we started coordinate proofs.  First we did a day of only using the distance formula.  (Yay more booklets!)

The next day we used only the slope formula to complete proofs.

2015-2016 Geometry INB Unit 8 (Measurement) [#MTBoS Blaugust]

It's #MTBoSBlaugust 2016!  I'm challenging myself to blog daily throughout August, and you can too.  Join the fun!

See last year's INB pages from this unit here.

This unit was completely overhauled from last year.  Last year, the majority of the notes were handwritten.  This year I made it more like my other notes.  Here is the table of contents:

I gave students two formula sheets.  The first is the Common Core exam formula sheet.  Since NYS gives students the same formula sheet for all three exams, I had students highlight the formulas that we use for Geometry.   The second formula sheet is something I adapted from a formula sheet I found somewhere online.  (It was so long ago now, I have no idea where I found it.)  It includes the area and perimeter formulas for 2D shapes.

We spent one day reviewing solving for perimeters and areas of 2D figures.  Students could definitely use more practice with these, but everything is so focused on 3D figures that it is hard to justify the time it would take.

The next day we worked on Solids.  Two big focuses of the Common Core curriculum are rotating 2D figures to create 3D figures (which is strange because it's so simple), and identifying cross-sections of 3D figures.  Since I don't have the skills to create diagrams of rotating figures, I used what I could find, and the diagrams seen on my page are from Math Bits Notebook (a great resource for Common Core Geometry information - it's very student friendly).  We had a fun activity for determining the cross section of solids using Play-Doh.  I previously blogged about this activity here.

We covered Volume of Prisms and Cylinders.  The examples here are from our textbook, and past Regents questions.

The same for Volume of Pyramids and Cones.

And the Volume of Spheres.

We finished the unit by exploring the area and volume of similar figures and solids.  Next year I want to change some of the examples to reflect how the Common Core exam asks about this topic. 

2015-2016 Geometry INB Unit 7 (Right Triangles) [#MTBoSBlaugust]

It's #MTBoSBlaugust 2016!  I'm challenging myself to blog daily throughout August, and you can too.  Join the fun!

See last year's INB pages from this unit here.

Here is the table of contents:

The only change I made from last year was to the Trigonometric Ratios page.  The top part of the page is a set of flaps.  The ratios are written under the flaps.  The examples are the same as they were the year before.  After the examples I added information and examples about the sine and cosine of complementary angles.  I used the lesson guide from the NYS modules for the notes and the examples.

 pdf

I am open to any ideas or suggestions you may have.  Feel free to comment below.

Editable word documents may be requested via email.

2015-2016 Geometry INB Unit 6 (Similarity) [#MTBoSBlaugust]

It's #MTBoSBlaugust 2016!  I'm challenging myself to blog daily throughout August, and you can too.  Join the fun!

See last year's INB pages from this unit here.

Here is the table of contents:

Two minor changes to this unit.  First, I expanded the "Proving Triangles Similar" page.  Note to self:  I definitely want to change this page for next year.  It's really just notes from the textbook that I have a love/hate relationship with.

I added a new page for "Indirect Measurement".  I blogged about the activity that accompanies these notes before.  I found some examples from the Internet and used the two regents questions on this topic.

2015-2016 Geometry INB Unit 5 (Polygons) [#MTBoSBlaugust]

It's #MTBoSBlaugust 2016!  I'm challenging myself to blog daily throughout August, and you can too.  Join the fun!

See last year's INB pages from this unit here.

Here is the table of contents:

I went to a conference in December, and decided to try this anticipation-reaction guide for parallelograms.  Students respond to the given statement before the lesson indicate whether they agree with the statement, and then after the lesson they reflect on their previous answer and their learning.  (Were they right, were they wrong, what did they learn, etc.)  The second half gives them a diagram of the parallelogram and asks them what they know/think to be true about the sides and angles, and then compare their answers with a partner.  This didn't go so well because it was new to my students.  I would like to try these more consistently next year.

Our parallelograms notes otherwise stayed the same, and I added in this partner activity.  The directions were projected on the board for students to either do the odd or even numbers, and then share and EXPLAIN their answers with their partner.  Their partner's job was to check their work.  Again, this is something I learned from the conference and would need to implement consistently for it go smoothly.  (I'm so glad I'm doing this reflection piece now to remind what to do differently for next year.)  These problems were adapted from a workbook I have.

I changed up my special parallelograms notes because my students were not fans of flaps and foldables.

Then I scoured the Internet to find some special parallelograms practice.  (The special parallelograms get no love.)  I finally found a packet of some sort and adapted some of the questions for a day of practice.

Then there were parallelogram proofs.  As booklets!

Then I concluded the notes for the unit by giving students an open-ended prompt to create a graphic organizer of their choice to summarize the properties of quadrilaterals.  Below is the example from my notebook.